Axisymmetric Horn¶
Problem description¶
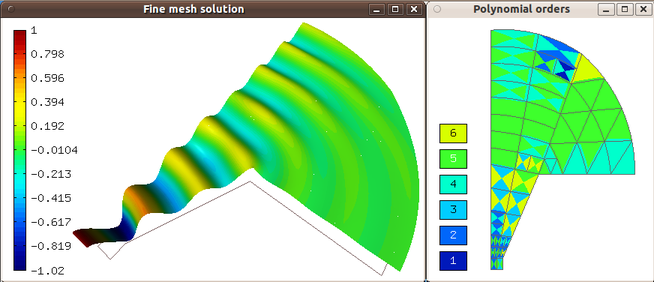
This example solves adaptively the pressure field in a 3D axisymmetric model of a harmonic acoustic horn. The geometry and initial mesh are shown below.
Equation solved:
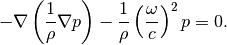
Boundary conditions are Dirichlet (prescribed pressure) on the bottom edge, zero Neumann (symmetry) on the left edge, Newton (matched boundary)
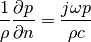
on the outlet arc and zero Neumann (wall) on the rest of the boundary. Here 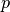 is pressure,
is pressure,
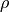 density of air,
density of air, 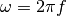 angular frequency, and
angular frequency, and  speed of sound. See
the main.cpp file for concrete values.
speed of sound. See
the main.cpp file for concrete values.