Gross-Pitaevski Equation¶

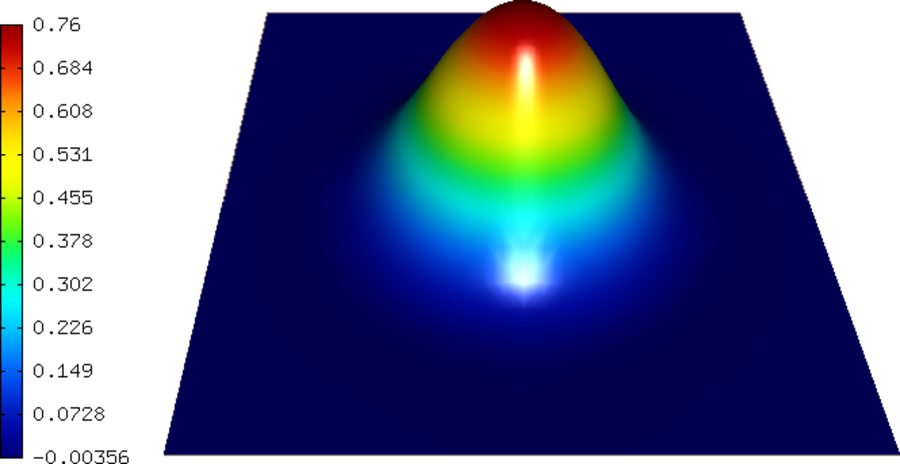
In this example we use the Newton’s method to solve the nonlinear complex-valued time-dependent Gross-Pitaevski equation. This equation describes the ground state of a quantum system of identical bosons using the Hartree–Fock approximation and the pseudopotential interaction model. For time-discretization one can use either the first-order implicit Euler method or the second-order Crank-Nicolson method.

Problem description¶

The computational domain is the square 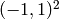$(-1,1)^2$ and boundary conditions are zero Dirichlet. The equation has the form

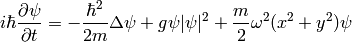
$i\hbar \frac{\partial \psi}{\partial t} = -\frac{\hbar^2}{2m} \Delta \psi + g \psi |\psi|^2 + \frac{m}{2} \omega^2 (x^2 + y^2) \psi$

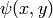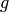where $\psi(x,y)$ is the unknown solution (wave function), $i$ the complex unit, $\hbar$ the Planck constant, $m$ the mass of the boson, $g$ the coupling constant (proportional to the scattering length of two interacting bosons) and 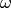$\omega$ the frequency.