Laminar Flame¶
Problem description¶
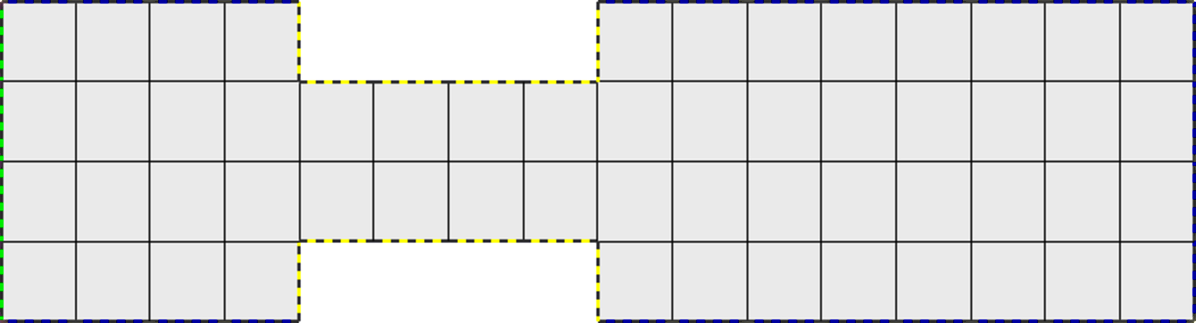
We will employ the Newton’s method to solve a nonlinear system of two parabolic equations describing a very simple flame propagation model (laminar flame, no fluid mechanics involved). The computational domain shown below contains in the middle a narrow portion (cooling rods) whose purpose is to slow down the chemical reaction:
The equations for the temperature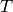 
and species concentration 
have the form
Boundary conditions are Dirichlet 
and 
on the inlet,
Newton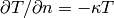 
on the cooling rods,
and Neumann 
, 
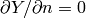elsewhere.
The objective of the computation is to obtain the reaction rate defined
by the Arrhenius law,
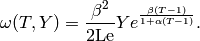
Here 
is the gas expansion coefficient in a flow with nonconstant density,
the non-dimensional activation energy, and
the Lewis number (ratio of diffusivity of heat and diffusivity
of mass). Both 
, 
and
, 
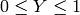are dimensionless and so is the time 
.
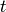Second-order BDF formula for time integration¶
Time integration is performed using a second-order implicit BDF formula
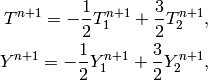
that is obtained using a combination of the following two first-order methods:
and
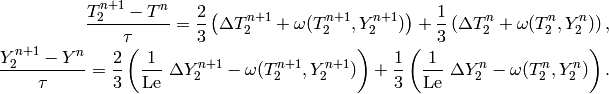
Problem parameters are chosen as
// Problem constants
const double Le = 1.0;
const double alpha = 0.8;
const double beta = 10.0;
const double kappa = 0.1;
const double x1 = 9.0;
Initial conditions¶
It is worth mentioning that the initial conditions for 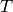and ,
// Initial conditions.
scalar temp_ic(double x, double y, scalar& dx, scalar& dy)
{ return (x <= x1) ? 1.0 : exp(x1 - x); }
scalar conc_ic(double x, double y, scalar& dx, scalar& dy)
{ return (x <= x1) ? 0.0 : 1.0 - exp(Le*(x1 - x)); }
are defined as exact functions:
// Set initial conditions.
t_prev_time_1.set_exact(&mesh, temp_ic); c_prev_time_1.set_exact(&mesh, conc_ic);
t_prev_time_2.set_exact(&mesh, temp_ic); c_prev_time_2.set_exact(&mesh, conc_ic);
t_prev_newton.set_exact(&mesh, temp_ic); c_prev_newton.set_exact(&mesh, conc_ic);
Here the pairs of solutions (t_prev_time_1, y_prev_time_1) and (t_prev_time_2, y_prev_time_2) correspond to the two first-order time-stepping methods described above. and (t_prev_newton, y_prev_newton) are used to store the previous step approximation in the Newton’s method.
Using Filters¶
The reaction rate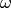 
and its derivatives are handled
via Filters:
// Filters for the reaction rate omega and its derivatives.
DXDYFilter omega(omega_fn, Tuple<MeshFunction*>(&t_prev_newton, &c_prev_newton));
DXDYFilter omega_dt(omega_dt_fn, Tuple<MeshFunction*>(&t_prev_newton, &c_prev_newton));
DXDYFilter omega_dc(omega_dc_fn, Tuple<MeshFunction*>(&t_prev_newton, &c_prev_newton));
Details on the functions omega_fn, omega_dt_fn, omega_dy_fn and the weak forms can be found in the file forms.cpp
Reinitialization of Filters¶
Notice the reinitialization of the Filters at the end of the Newton’s loop. This is necessary as the functions defining the Filter have changed:
// Set current solutions to the latest Newton iterate
// and reinitialize filters of these solutions.
Solution::vector_to_solutions(newton.get_sln_vector(), Tuple<Space *>(&tspace, &cspace),
Tuple<Solution *>(&t_prev_newton, &c_prev_newton));
omega.reinit();
omega_dt.reinit();
omega_dc.reinit();
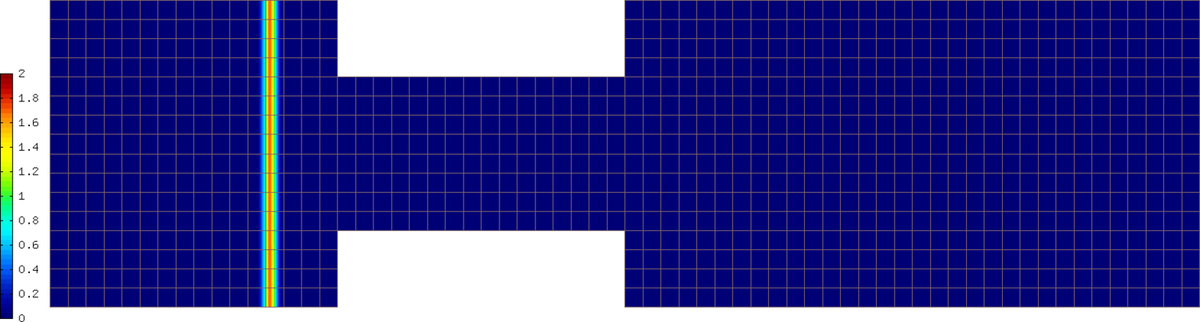
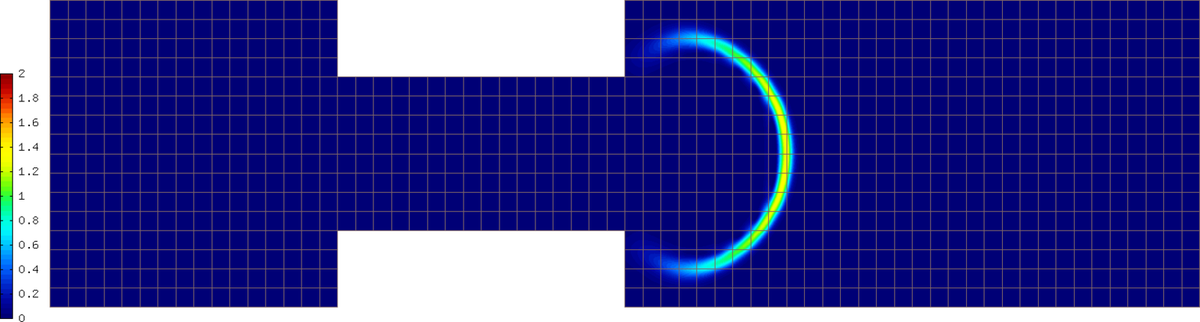
Visualization of a Filter¶
Also notice the visualization of a Filter:
// Visualization.
DXDYFilter omega_view(omega_fn, Tuple<MeshFunction*>(&t_prev_newton, &c_prev_newton));
rview.set_min_max_range(0.0,2.0);
char title[100];
sprintf(title, "Reaction rate, t = %g", current_time);
rview.set_title(title);
rview.show(&omega_view);
